Power Law,or the Law of Scaling.

Nobody can directly say who invented Power Law, but it draws influence from Issacc Netwon’s works extensively.

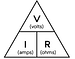
In electronics, the Power Law refers to the relationship between power, voltage, and current in an electrical circuit. The law helps us calculate the power consumed or delivered by a component in the circuit, such as a resistor, using the following formulas.

Basic Formula

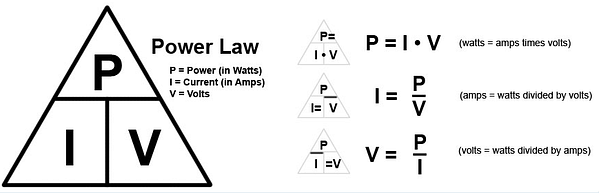
The basic formula for power (P) in an electrical circuit is:P=V×IP = V \times IP=V×I

Where:

• P is the power (measured in watts, W),
• V is the voltage across the component (measured in volts, V),
• I is the current flowing through the component (measured in amperes, A).

Derived Formulas Using Ohm’s Law

Since Ohm’s Law states that V=I×RV = I \times RV=I×R, we can substitute this into the power formula to derive two additional forms:

1. Power in terms of current and resistance:P=I2×RP = I^2 \times RP=I2×R
   • Here, power is proportional to the square of the current (I2I^2I2) and the resistance (RRR).
2. Power in terms of voltage and resistance:P=V2RP = \frac{V^2}{R}P=RV2​
   • Here, power is proportional to the square of the voltage (V2V^2V2) and inversely proportional to the resistance (RRR).

Understanding the Relationships

• Direct Relationship Between Power and Voltage/Current: If you increase the voltage or current in a circuit while keeping the other factors constant, the power consumed by the circuit will increase.
• Current and Resistance: If the resistance is constant, increasing the current leads to an increase in power, which is why the formula P=I2×RP = I^2 \times RP=I2×R is often used in scenarios like heating, where power dissipation (in the form of heat) depends on the current.
• Voltage and Resistance: Similarly, if resistance is constant, increasing the voltage across a component increases the power dissipated by it, as described by P=V2RP = \frac{V^2}{R}P=RV2​.

Practical Applications

• Power Consumption: Knowing the power consumed by different components helps in designing circuits to ensure they operate within safe limits. Exceeding the power rating of a component can cause it to overheat or get damaged.
• Power Supply Design: In power supply design, engineers use these formulas to calculate the necessary ratings of components like resistors and transistors to ensure efficient and safe operation.
• Heat Dissipation: Since power dissipation often leads to heat, understanding power relationships is crucial in managing thermal performance in electronic devices.

Examples

1. Example 1: If a resistor has a resistance of 10Ω \Omega10Ω and a current of 2A flows through it, the power dissipated by the resistor can be calculated as:P=I2×R=22×10=4×10=40 WP = I^2 \times R = 2^2 \times 10 = 4 \times 10 = 40 \, WP=I2×R=22×10=4×10=40W
2. Example 2: If you apply 12V across the same 10Ω \Omega10Ω resistor, the power dissipated would be:P=V2R=12210=14410=14.4 WP = \frac{V^2}{R} = \frac{12^2}{10} = \frac{144}{10} = 14.4 \, WP=RV2​=10122​=10144​=14.4W

In summary, the Power Law in electronics helps us understand how power is related to voltage, current, and resistance, enabling the design of safe and efficient electrical circuits.